(Photo: pixabay.com)

Pi is good, Tau is better

Everyone knows the circular number Pi (π), but do you know the mathematical constant Tau (τ)? Tau describes the ratio of the circumference of a circle to its radius (τ = U/r). Or more simply, tau is the circumference of the unit circle (radius = 1).

... By Markus Fleschutz 🕓 March 17, 2019

In contrast to tau, pi describes the ratio of circumference to diameter of the circle (π = U/d). Problem with this: Circle formulas never use the diameter, but always the radius. This 'birth defect' led to the fact that in many formulas "2π" occurs, which corresponds exactly to tau. The exact value of tau is:

τ = 6.283185307179586476925286766559...

Using tau instead of pi simplifies many formulas and makes it easier to get started with circular geometry. Here are a few examples:

Circumference

The calculation of the circumference (U), i.e. the length of a circle line is calculated from the circle radius (r) alone as follows:

U = τ - r

Example: a circle with a radius of 7 cm has a circumference of 43.9... cm.

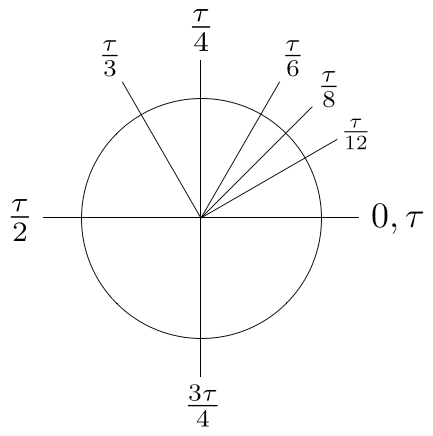
With Tau, the subdivision of the circumference of a circle into semicircles, quarter circles, eighth circles, etc. is completely simple and natural:

Circular area

The calculation of the area of the circle (A) results from the radius of the circle (r) alone:

A = ½τ - r²

Example: a circle with a radius of 7 cm has an area of 153.9... cm.

Circle sector

A circle sector (also circle sector) is in geometry the partial area of a circle surface, which is limited by a circle arc and two circle radii. A circle sector is defined by radius (r) and the angle (α).

Arc length: b = τ - r - (α / 360°)

Area: A = ½τ - r² - (α / 360°) or A = (b - r) / 2

Circumference: U = 2-r + τ-r-(α / 360°)

Actually quite simple: take the formula for circumference or area of a circle and multiply it by the ratio of the angle (α) to the full circle (360°). For the circumference, of course, add the two radii of the circle.

Circular ring

A circular ring is formed by two concentric (same center) circles of different sizes, subtracting the smaller from the larger. A circular ring is determined by radius r1 (the larger one) as well as radius r2 (the smaller one).

Circumference: U = τ - (r1 + r2)

Area: A = ½τ - (r1² - r2²)

Volume of a sphere

The volume (V) of a sphere is determined by the radius (r) as follows:

V = ⅔τ - r³

Example: a sphere with a radius of 2 cm has a volume of 33.5... cm³.

Surface area of a sphere

The surface area (O) of a sphere is determined by the radius (r) as follows:

O = 2τ - r²

Example: a sphere with a radius of 2 cm has a surface area of 50.3... cm².

Volume of a cylinder

The volume (V) of a cylinder is determined from radius (r) and height (h):

V = ½τ - r² - h

Example: a cylinder with a radius of 5 cm and a height of 10 cm has a volume of 785.4... cm³.

Surface area of a cylinder

The surface area (O) of a cylinder is also determined from radius (r) and height (h):

O = τ - r² + τ - r - h

Example: a cylinder with a radius of 5 cm and a height of 8 cm has a surface area of 408.4... cm².

Volume of an ellipsoid

An ellipsoid is the 3-dimensional equivalent of an ellipse. The volume (V) of an ellipsoid is determined by the semi-axes (a), (b), and (c):

V = ⅔τ - a - b - c

Radiant

The radian (unit symbol "rad") is an angular measure in which the angle is given by the length of the corresponding arc in the unit circle. Because of the consideration of the circular arc for the indication of the angle, the indication "in radian measure" is also called arc angle.

The range of values in the unit circle is between 0 rad and τ rad (corresponding to 360°, also called full angle or full circle). In many calculations in physics and mathematics, the radian is the most useful angular measure (for example, for angular velocity and sine and cosine).

Here is the conversion radian ↔ degree for the unit circle:

rad = deg - τ/360
deg = rad - 360/τ

Example: an angle of 90° corresponds to 1.5707... rad for the unit circle.

Sum of all interior angles

In a square (whether square, rectangle, rhombus, trapezoid, or parallelogram), the sum of all interior angles is always 360° or τ rad:

α + β + γ + δ = 360°.

In a triangle (no matter what shape), the sum of all interior angles is always 180° or ½τ rad:

α + β + γ = 180°

Sine and cosine

The sine of an angle (θ) is the ratio of the length of the opposite cathetus (cathetus opposite the angle) to the length of the hypotenes